

e-mail :

This document continues the investigation of special categories (If / Then constants), and compares crystals with organisms.
Crystals and Organisms, Shape, Symmetry and Promorph.
Sequel to the investigation of some (intrinsic) shapes of two-dimensional crystals regarding their relationship to intrinsic point symmetry and promorph.
Equilateral Triangle (Promorphs)
The Vector Rosette of Actual Growth of a regularly-triangular crystal, i.e. of any two-dimensional crystal having as its intrinsic shape (when fully developed) the Equilateral Triangle (which itself has D3 symmetry) and possessing whatever (possible) intrinsic (point) symmetry, is as follows (blue lines) :
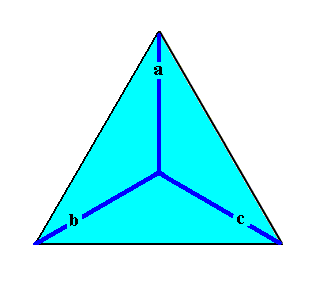
This Vector Rosette, viz. a vector rosette of a regularly-triangular two-dimensional crystal, in fact consists of three vectors, a, b, c, each originating in the center of the crystal and ending up at a corner. As such it encodes the crystal's shape.
REMARK :
As in the previous document, we will often refer to a "regularly-triangular" crystal or shape. So the reference is to the equilateral triangle. In the sequel we will use the term "triangular" while this term should be taken to mean "regularly-triangular", unless indicated otherwise.
As we saw in the previous document, an intrinsically triangular crystal shape (with respect to two-dimensional crystals) is supported by the following plane groups :
P3m1, P3, Pm, Pg, Cm and P1.
See also the table in the previous document where, in addition to the supporting plane groups, the corresponding point groups are indicated, and the number of crystallographic Forms needed to construct a triangular crystal in each case (of point symmetry).
We will now consider several possible cases of the given example of a triangular two-dimensional crystal as depicted just above, with respect to possible promorphs.
D3 symmetry.

Figure above : Symmetry elements of a two-dimensional intrinsically triangular crystal with D3 intrinsic symmetry : Three mirror lines ( m1 , m2 , m3 ), and one 3-fold rotation axis at their point of intersection.
One crystallographic Form is needed to conceptually construct a triangular D3 crystal. See next Figure.
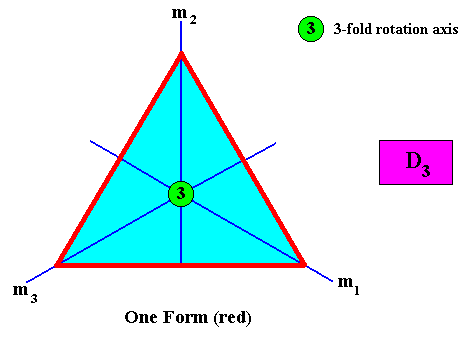
Figure above : Only one crystallographic Form is needed to construct a triangular D3 two-dimensional crystal : One initially given crystal face implies two others in virtue of the 3-fold rotation axis, resulting in one Form consisting of three faces, together making up the triangular crystal.
D3 symmetry and three antimers.

Figure above : The three antimers (blue, green, yellow) of a two-dimensional triangular crystal with intrinsic D3 symmetry. Radial (R) and interradial (IR) directions indicated.
And the Vector Rosette of Actual Growth added :

Figure above : The three antimers (blue, green, yellow) of a two-dimensional triangular crystal with intrinsic D3 symmetry. Radial (R) and interradial (IR) directions indicated. Vector Rosette of Actual Growth added. The vectors a, b, and c are equivalent.
The next Figure depicts and names the promorph of our two-dimensional triangular D3 crystal. It is an equilateral triangle, and as such the two-dimensional analogue of the regular three-fold pyramid. As promorph it is the two-dimensional analogue of an instance of the (three-dimensional) Homostaura Anisopola triactinota.
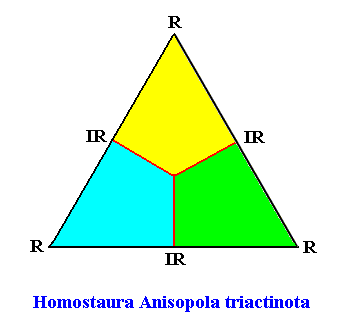
Figure above : Promorph of the above discussed two-dimensional triangular D3 crystal. The three antimers are indicated (blue, green, yellow).
The next Figure gives the three-dimensional analogue of the promorph of the previous Figure.
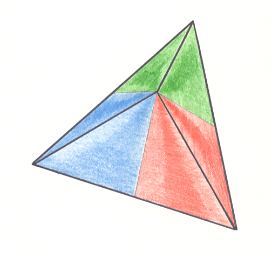
Figure above : The regular 3-fold pyramid (oblique top-view) representing the Homostaura Anisopola triactinota (as a category of three-dimensional promorphs). The three antimers are indicated by colors.
C3 symmetry.
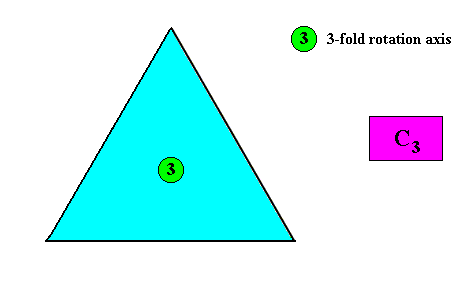
Figure above : The only symmetry element of a two-dimensional triangular C3 crystal : a three-fold rotation axis in the center of the triangle.
One crystallographic Form is needed to conceptually construct a triangular C3 crystal. See next Figure.
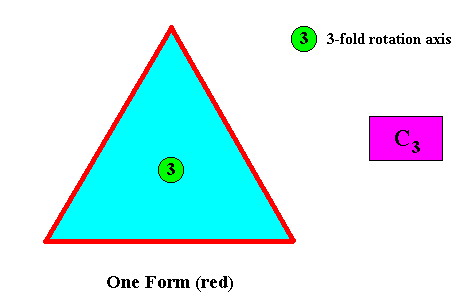
Figure above : Only one crystallographic Form is needed to construct a triangular C3 two-dimensional crystal : One initially given crystal face implies two others in virtue of the 3-fold rotation axis, resulting in one Form consisting of three faces, together making up the triangular crystal.
C3 symmetry and three antimers.
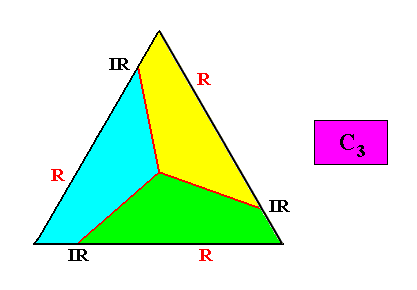
Figure above : The three antimers (blue, green, yellow) of a two-dimensional triangular crystal with intrinsic C3 symmetry. Radial (R) and interradial (IR) directions indicated.
And the Vector Rosette of Actual Growth added :
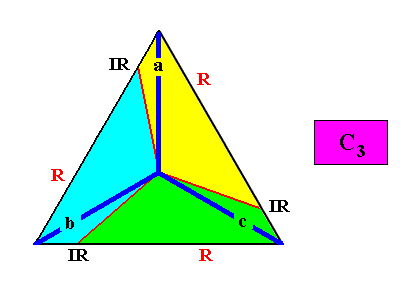
Figure above : The three antimers (blue, green, yellow) of a two-dimensional triangular crystal with intrinsic C3 symmetry. Radial (R) and interradial (IR) directions indicated. Vector Rosette of Actual Growth added. The vectors a, b, and c are equivalent.
The next Figure depicts and names the promorph of our two-dimensional triangular C3 crystal. It is a regular three-fold gyroid polygon (trigonal gyroid polygon), and as such the two-dimensional analogue of the regular three-fold gyroid pyramid (trigonal gyroid pyramid). As promorph it is the two-dimensional analogue of an instance of the (three-dimensional) Homogyrostaura trimera.
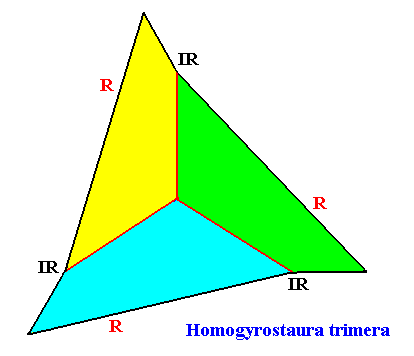
Figure above : Promorph of the above discussed two-dimensional triangular C3 crystal. The three antimers are indicated (blue, green, yellow).
The next Figure gives the three-dimensional analogue of the promorph of the previous Figure.
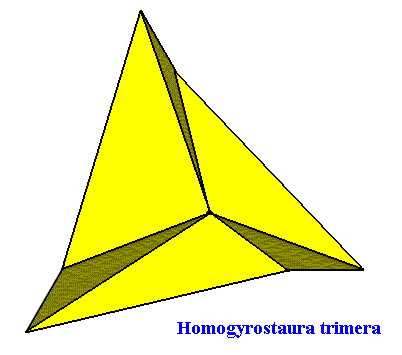
Figure above : Slightly oblique top-view of the trigonal (3-fold) gyroid pyramid representing the Homogyrostaura trimera (as a category of three-dimensional promorphs).
D1 symmetry.
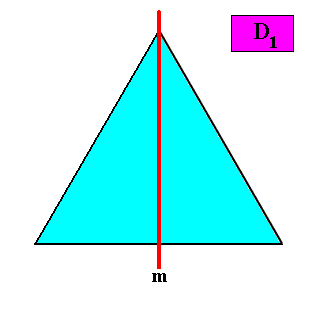
Figure above : The only symmetry element of a two-dimensional triangular D1 crystal is a mirror line ( m ).
Two crystallographic Forms are needed to conceptually construct a triangular D1 crystal. See next Figure.
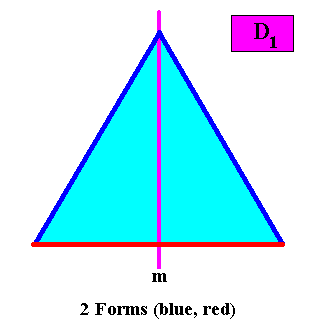
Figure above : Two crystallographic Forms are needed to construct a triangular D1 two-dimensional crystal : One initially given crystal face (blue), not perpendicular or parallel to the mirror line, implies one other face (blue) in virtue of that mirror line, resulting in one open Form (blue) consisting of two faces. Another initially given face (red), perpendicular to the mirror line, does not imply other faces, so this one face constitutes an open Form (red). The two Forms together make up the whole triangular crystal.
A crystal with intrinsic D1 symmetry can in principle have any number (> 1) of antimers depending on the geometry of the motif as translation-free residue. We will discuss several examples.
D1 symmetry and two antimers.
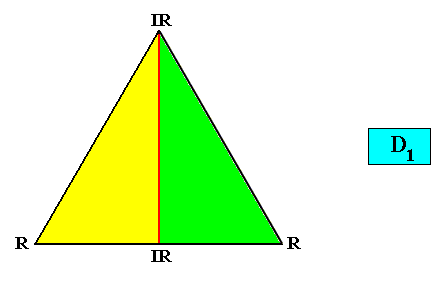
Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and two antimers (green, yellow). Radial (R) and interradial (IR) directions indicated.
And the Vector Rosette of Actual Growth added :
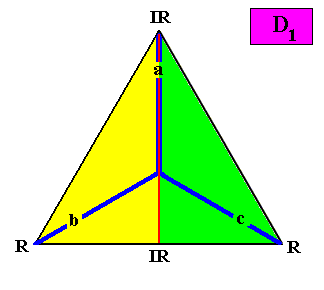
Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and two antimers (green, yellow). Radial (R) and interradial (IR) directions indicated. Vector Rosette of Actual Growth added. The vectors b and c are equivalent.
The next Figure depicts and names the promorph of our two-dimensional triangular D1 crystal. It is an isosceles triangle and as such the two-dimensional analogue of half a rhombic pyramid. As promorph it is the two-dimensional analogue of an instance of the (three-dimensional) Allopola Zygopleura eudipleura.
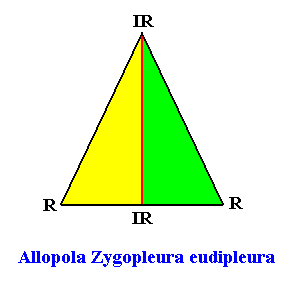
Figure above : Promorph of the above discussed two-dimensional triangular D1 crystal. The two antimers are indicated (green, yellow).
D1 symmetry with four antimers.
Interradial configuration of the four antimers.
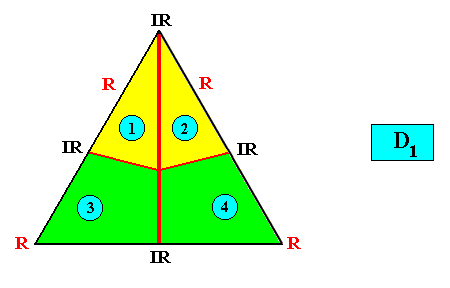
Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and four antimers (green, yellow, and indicated by numerals). Interradial configuration. Radial (R) and interradial (IR) directions indicated.
And the Vector Rosette of Actual Growth added :
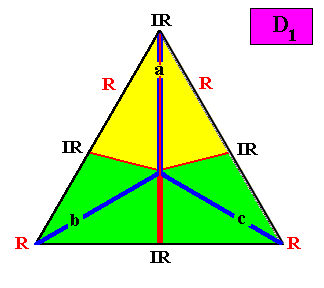
Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and four antimers (green, yellow). Interradial configuration. Radial (R) and interradial (IR) directions indicated. Vector Rosette of Actual Growth added. The vectors b and c are equivalent.
The symmetry of our crystal only demands that the configuration and shapes of the four antimers as a whole comply with the one mirror line of the crystal. And this means that the common point of the antimers can be positioned anywhere on the mirror line (which is vertical in the present case), dependent on the geometry of the motif as translation-free residue. The next Figure illustrates this freedom of positioning of the common point.
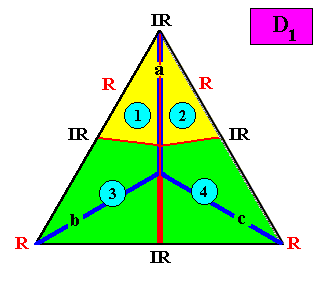
Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and four antimers (green, yellow, and indicated by numerals). Vector Rosette of Actual Growth added. Alternative position of common point.
The next Figure depicts and names the promorph of our two-dimensional triangular D1 crystal with an interradial configuration of its four antimers. It is an isosceles trapezium and as such the two-dimensional analogue of a trapezoid pyramid. As promorph it is the two-dimensional analogue of an instance of the (three-dimensional) Allopola Zygopleura Eutetrapleura interradialia.

Figure above : Promorph of the above discussed two-dimensional triangular D1 crystal. The four antimers are indicated (green, yellow).
Radial configuration of the four antimers.
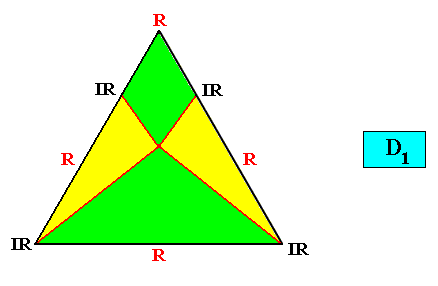
Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and four antimers (green, yellow). Radial configuration. Radial (R) and interradial (IR) directions indicated.
And the Vector Rosette of Actual Growth added :
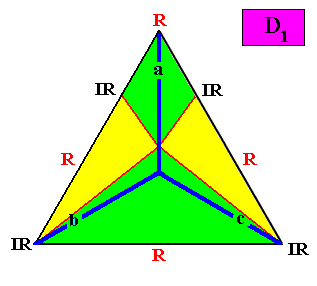
Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and four antimers (green, yellow). Radial configuration. Radial (R) and interradial (IR) directions indicated. Vector Rosette of Actual Growth added. The vectors b and c are equivalent.
The next Figure depicts and names the promorph of our two-dimensional triangular D1 crystal with radial configuration of its four antimers. It is a bi-isosceles triangle and as such the two-dimensional analogue of a bi-isosceles pyramid. As promorph it is the two-dimensional analogue of an instance of the (three-dimensional) Allopola Zygopleura Eutetrapleura radialia.
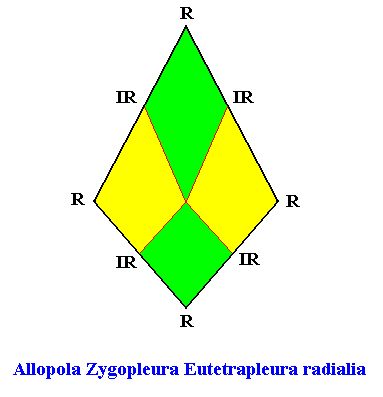
Figure above : Promorph of the above discussed two-dimensional triangular D1 crystal. The four antimers are indicated (green, yellow).
D1 symmetry and three antimers.
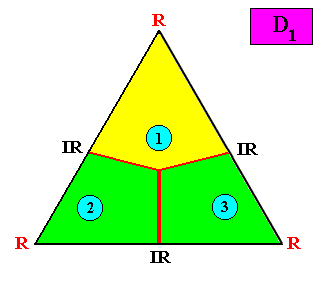
Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and three antimers (green, yellow, and indicated by numerals).
And the Vector Rosette of Actual Growth added :
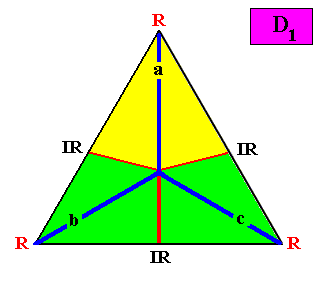
Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and three antimers (green, yellow). Vector Rosette of Actual Growth added. The vectors b and c are equivalent.
The symmetry of our crystal only demands that the configuration and shapes of the three antimers as a whole comply with the one mirror line of the crystal. And this means that the common point of the antimers can be positioned anywhere on the mirror line (which is vertical in the present case), dependent on the geometry of the motif as translation-free residue. The next Figure illustrates this freedom of positioning of the common point.

Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and three antimers (green, yellow, and indicated by numerals). Vector Rosette of Actual Growth added. Alternative position of common point.
The next Figure depicts and names the promorph of our two-dimensional triangular D1 crystal with three antimers. It is half a six-fold amphitect polygon, and as such the two-dimensional analogue of half a six-fold amphitect pyramid. As promorph it is the two-dimensional analogue of an instance of the (three-dimensional) Allopola Amphipleura triamphipleura.
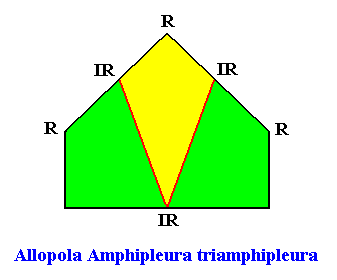
Figure above : Promorph of the above discussed two-dimensional triangular D1 crystal. The three antimers are indicated (green, yellow).
D1 symmetry and five antimers.
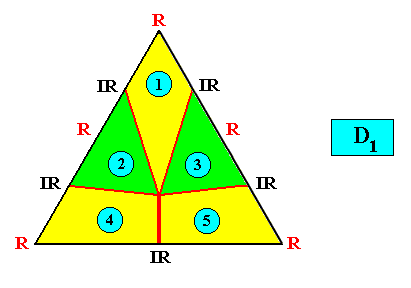
Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and five antimers (green, yellow, and indicated by numerals).
And the Vector Rosette of Actual Growth added :
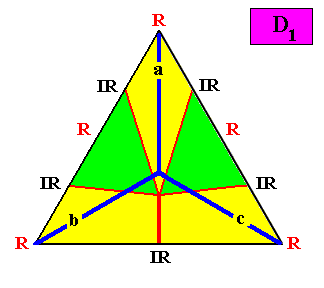
Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and five antimers (green, yellow). Vector Rosette of Actual Growth added. The vectors b and c are equivalent.
The next Figure depicts and names the promorph of our two-dimensional triangular D1 crystal with five antimers. It is half a ten-fold amphitect polygon, and as such the two-dimensional analogue of half a ten-fold amphitect pyramid. As promorph it is the two-dimensional analogue of an instance of the (three-dimensional) Allopola Amphipleura pentamphipleura.
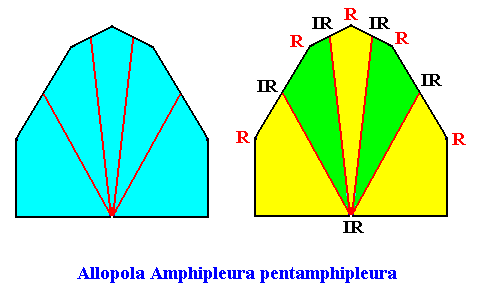
Figure above : Promorph of the above discussed two-dimensional triangular D1 crystal. In the right image the five antimers are indicated by colors (green, yellow).
D1 symmetry and six antimers.

Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and six antimers (green, yellow, and indicated by numerals).
And the Vector Rosette of Actual Growth added :
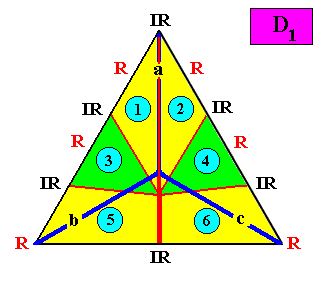
Figure above : A two-dimensional intrinsically triangular crystal with intrinsic D1 symmetry and six antimers (green, yellow, and indicated by numerals). Vector Rosette of Actual Growth added. The vectors b and c are equivalent.
The next Figure depicts and names the promorph of our two-dimensional triangular D1 crystal with six antimers. It is half a twelve-fold amphitect polygon, and as such the two-dimensional analogue of half a twelve-fold amphitect pyramid. As promorph it is the two-dimensional analogue of an instance of the (three-dimensional) Allopola Amphipleura hexamphipleura.

Figure above : Promorph of the above discussed two-dimensional triangular D1 crystal. In the right image the six antimers are indicated by colors (green, yellow).
C1 symmetry and no antimers.
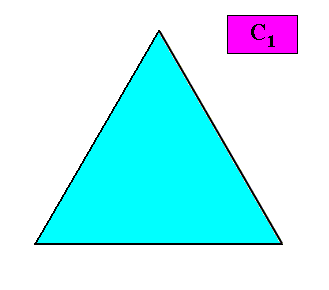
Figure above : A (two-dimensional) C1 crystal does not have any symmetry elements (with respect to point symmetry).
Three crystallographic Forms are needed to conceptually construct a two-dimensional triangular crystal with intrinsic C1 symmetry. See next Figure.

Figure above : Three crystallographic Forms are needed to construct a triangular two-dimensional C1 crystal : Any intially given crystal face does not imply other faces. So each face is itself already a crystallographic Form. Evidently three such Forms are needed to construct an equilateral triangle , and thus the whole triangular C1 crystal.
C1 crystals cannot have genuine antimers. See next Figures.
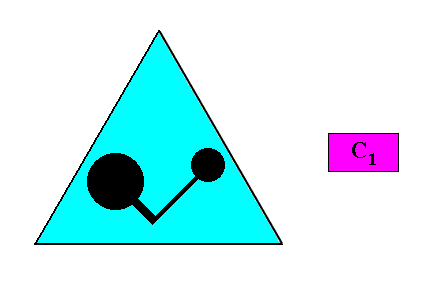
Figure above : A two-dimensional intrinsically triangular C1 crystal.
A motif (black) is inserted to express the crystal's C1 symmetry.
And with the Vector Rosette of Actual Growth added :
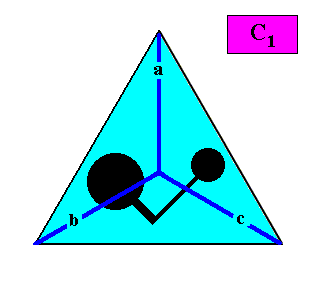
Figure above : A two-dimensional intrinsically triangular C1 crystal. A motif (black) is inserted to express the crystal's C1 symmetry. Vector Rosette of Actual Growth added. All vectors a, b, c, are unique (i.e. non-equivalent).
The next Figure (two images) gives the possible promorphs (and their names) of our triangular C1 crystal. The promorph is either (left image) half an isosceles triangle (and, equivalently, a quarter of a rhombus), and is as such the two-dimensional analogue of a quarter of a rhombic pyramid, or (right image) an irregular triangle, indicating two unequal antimers (yellow, green), and is as such the two-dimensional analogue of an irregular pyramid (or, equivalently, a 1-fold pyramid).
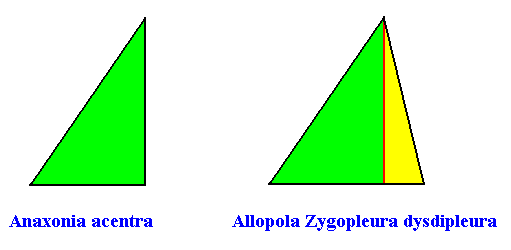
This concludes our investigation concerning the relation of intrinsic triangular (2-dimensional) crystal shape to intrinsic point symmetry and to promorph.
In the next document we will investigate the sixth and last shape from our above given list : rectangle, square, parallelogram, rhombus, equilateral triangle, regular hexagon.
e-mail : 
To continue click HERE for further study of the Theory of Layers, Part XIII.
e-mail : 