

e-mail :

This document continues the investigation of special categories (If / Then constants), and compares crystals with organisms.
Crystals and Organisms, Shape, Symmetry and Promorph.
Sequel to the investigation of some (intrinsic) shapes of two-dimensional crystals regarding their relationship to intrinsic point symmetry and promorph.
Amphitect Octagon
We will now investigate two-dimensional crystals with an intrinsic shape according to an amphitect octagon as to their possible promorphs, but, as in the foregoing, limiting ourselves to holomorphic crystals, which means that we will only investigate crystals of which their intrinsic symmetry is the same as the symmetry of their intrinsic shape. In the present case this means that our amphitect octagonal crystals will have D2 intrinsic symmetry (while in other cases -- meromorphic crystals -- this symmetry could be either according to D1 , or C2 , or C1 , depending on the crystal's internal structure).

Figure above : Microscopic view of a two-dimensional amphitect octagonal D2 crystal consisting of the periodic stacking of rectangular building blocks, explicitly provided with D2 motifs. These D2 motifs represent the translation-free residue of the crystal (all the same whether it belongs to the plane group P2mm, P2mg, P2gg or C2mm), and in this example the residue has eight antimers. So the crystal of this example itself has eight antimers and is thus eupromorphic (because the eight antimers are already evident in the crystal's intrinsic shape). Because the building blocks are in fact very small (i.e. in crystals they have microscopic dimensions), all the crystal faces are macroscopically smooth. The center of the crystal is highlighted (green).
By removing the lattice lines (indicating building blocks) and motifs, we obtain a macroscopic view of the above crystal :
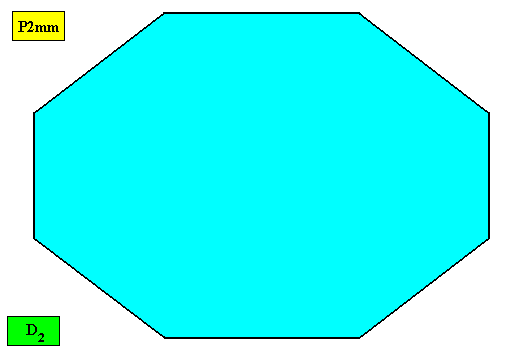
Figure above : Macroscopic view of the two-dimensional amphitect octagonal D2 crystal of the previous Figure.
The pattern of symmetry elements (with respect to the point symmetry) of our amphitect octagonal D2 two-dimensional crystal is given in the next Figure.
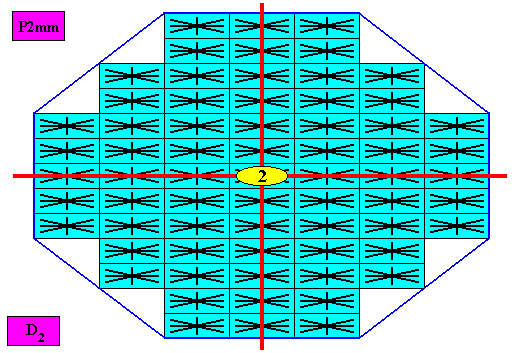
Figure above : Pattern of symmetry elements of the above given amphitect octagonal D2 two-dimensional crystal. Its consists of two mirror lines (red) perpendicular to each other, and a 2-fold rotation axis (small yellow ellipse) at their point of intersection.
Three crystallographic Forms are needed to construct the outline of the amphitect octagonal crystal, as the next Figure shows.
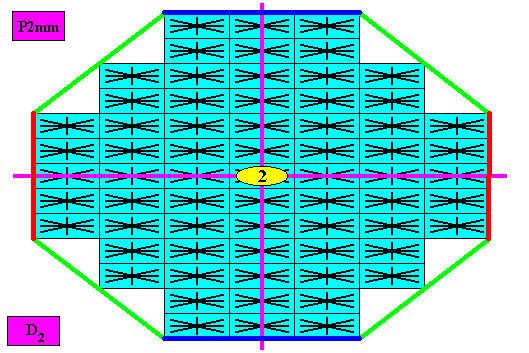
Figure above : Three crystallographic Forms (green, red, dark blue) are needed to construct the faces of our amphitect octagonal D2 two-dimensional crystal : An initially given oblique face (green, not parallel to either mirror line) implies three more faces in virtue of the symmetry elements, resulting in one Form consisting of four faces (green). This is a closed Form, and as such can represent a crystal. However, this crystal has the shape of a rhombus, and not that of an amphitect octagon. Two more Forms are needed : An initially given face (red) parallel to one of the mirror lines, implies one more face in virtue of the symmetry elements, resulting in a Form (red) consisting of two parallel faces. Another initially given face (dark blue) parallel to the other mirror line also implies one more face, resulting in yet another Form (dark blue) consisting of two parallel faces. These three Forms combined finally yield our amphitect octagonal crystal.
Possible a n t i m e r c o n f i g u r a t i o n s for holomorphic amphitect octagonal two-dimensional crystals.
Eight antimers.
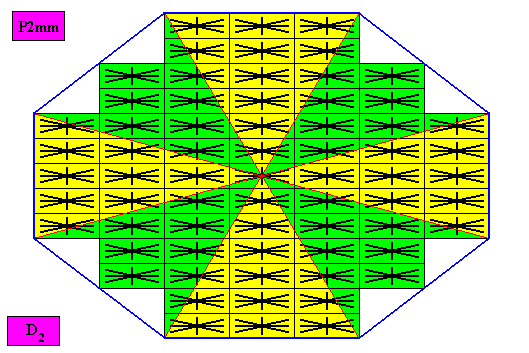
Figure above : An amphitect octagonal D2 two-dimensional crystal. The case of e i g h t similar antimers (green, yellow). Note the correspondence between the morphology of the (microscopic) motif (as translation-free residue of the crystal) and the arrangement of the (macroscopic) antimers of the crystal. In this way the promorph, and in particular the number of antimers, is based on the morphology of the translation-free residue of the crystal. This residue is explicitly given in the form of a D2 motif (black) inside each rectangular building block. It is -- or represents -- an atomic configuration such that eight antimers can be distinguished in it. The crystal is eupromorphic because its intrinsic shape suggests eight antimers, which indeed are present.
By removing lattice lines and motifs we obtain a macroscopic view of the crystal :
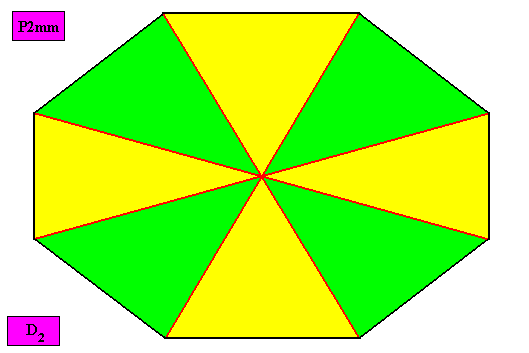
Figure above : Macroscopic view of the amphitect octagonal D2 two-dimensional crystal of the previous Figure with eight antimers (green, yellow).
The promorph of the above amphitect octagonal eupromorphic crystal is (as two-dimensional analogue) belonging to the Autopola Oxystaura octophragma. This promorph is depicted in the next Figure.
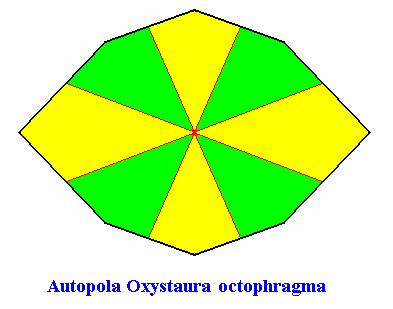
Figure above : The promorph of the amphitect octagonal crystal with eight antimers. It is an 8-fold amphitect polygon (amphitect octagon) and as such the two-dimensional analogue of the 8-fold amphitect pyramid, which represents the promorph of corresponding three-dimensional crystals or other objects.
Four antimers, radial configuration.
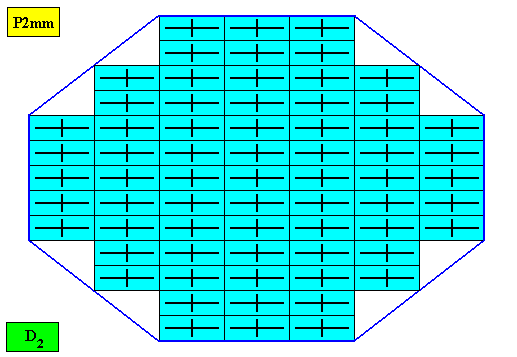
Figure above : An amphitect octagonal D2 two-dimensional crystal. Its D2 motifs (black) have four radially arranged antimers. Microscopic view.
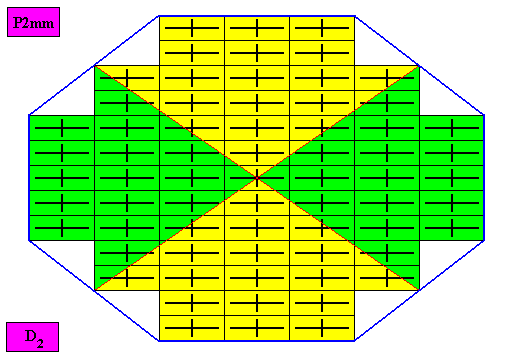
Figure above : The amphitect octagonal D2 two-dimensional crystal of the previous Figure. The case of f o u r similar antimers (green, yellow). Note the correspondence between the morphology of the (microscopic) motif (as translation-free residue) and the arrangement of the (macroscopic) antimers of the crystal. In this way the promorph, and in particular the number of antimers is based on the morphology of the translation-free residue of the crystal. This residue is explicitly given in the form of a D2 motif (black) inside each rectangular building block. It is -- or represents -- an atomic configuration such that four antimers can be distinguished in it. The four antimers of this (microscopic) atomic configuration are radially arranged (perpendicular directional cross axes passing through antimers), which will reflect itself in the promorph of the crystal. The crystal is non-eupromorphic because its intrinsic shape suggests eight antimers, while in fact there are only four of them.
By removing lattice lines and motifs we obtain a macroscopic view of the crystal :
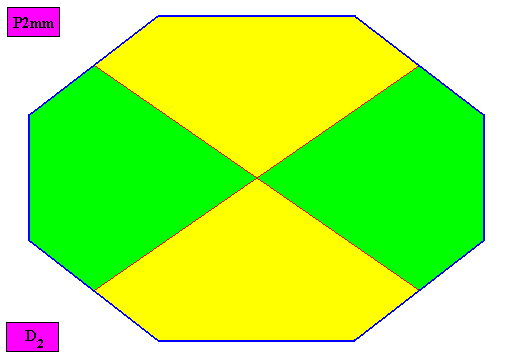
Figure above : Macroscopic view of the amphitect octagonal D2 two-dimensional crystal of the previous Figure with four radially arranged antimers (green, yellow).
The promorph of the above amphitect octagonal non-eupromorphic crystal is (as two-dimensional analogue) belonging to the Autopola Orthostaura Tetraphragma radialia. This promorph is depicted in the next Figure.
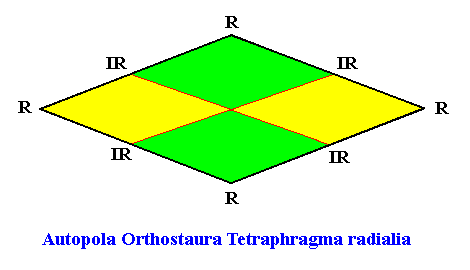
Figure above : The promorph of the amphitect octagonal crystal with four antimers. It is a 4-fold amphitect polygon (rhombus) and as such the two-dimensional analogue of the rhombic pyramid, which represents the promorph of corresponding three-dimensional crystals or other objects. Note the difference in shape between this promorph (rhombus, amphitect tetragon) and that of the crystal (amphitect octagon) of which it is the promorph.
As noted earlier (previous document) the above promorph (Autopola Orthostaura Tetraphragma radialia) can be expressed in a more general way than was done in the previous Figure, to express the possible non-congruity (and thus non-equality) of the antimers (only two by two equal). In the previous Figure, which depicted a representation of this promorph, the antimers were (drawn) equal. The more general representation is depicted in the next Figure.

Figure above : Slightly different representation of the promorph (Autopola Orthostaura Tetraphragma radialia) of the amphitect octagonal crystal with four antimers in radial configuration. It also is a 4-fold amphitect polygon (rhombus), but now expresses the unequality of the antimers as they are in the above amphitect octagonal crystal.
Four antimers, interradial configuration.
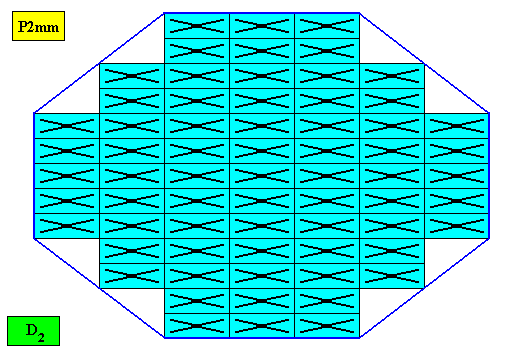
Figure above : An amphitect octagonal D2 two-dimensional crystal. Its D2 motifs (black) have four interradially arranged antimers. Microscopic view.
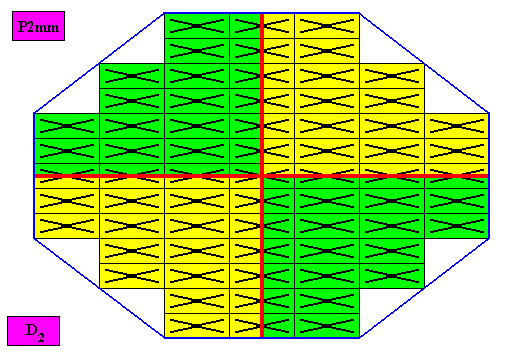
Figure above : The amphitect octagonal D2 two-dimensional crystal of the previous Figure. The interradial case of f o u r congruent (two by two equal) antimers (green, yellow). Note the correspondence between the morphology of the (microscopic) motif (as translation-free residue) and the arrangement of the (macroscopic) antimers of the crystal. In this way the promorph, and in particular the number of antimers is based on the morphology of the translation-free residue of the crystal. This residue is explicitly given in the form of a D2 motif (black) inside each rectangular building block. It is -- or represents -- an atomic configuration such that four congruent antimers can be distinguished in it. The four antimers of this (microscopic) atomic configuration are interradially arranged (perpendicular directional cross axes lying between antimers), which will reflect itself in the promorph of the crystal. The crystal is non-eupromorphic because its intrinsic shape suggests eight antimers, while in fact there are only four of them.
By removing lattice lines and motifs we obtain a macroscopic view of the crystal :
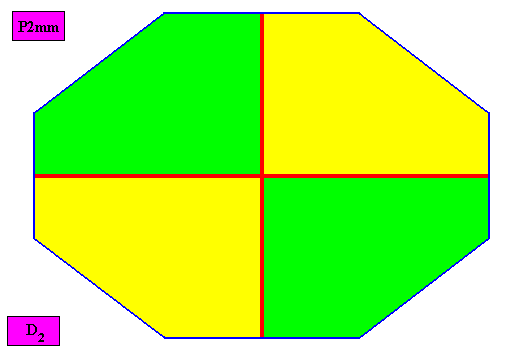
Figure above : Macroscopic view of the amphitect octagonal D2 two-dimensional crystal of the previous Figure with four interradially arranged antimers (green, yellow).
The promorph of the above amphitect octagonal non-eupromorphic crystal is (as two-dimensional analogue) belonging to the Autopola Orthostaura Tetraphragma interradialia. This promorph is depicted in the next Figure.
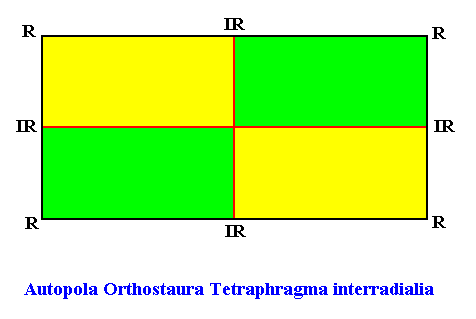
Figure above : The promorph of the amphitect octagonal crystal with four antimers. It is a rectangle and as such the two-dimensional analogue of the rectangular pyramid, which represents the promorph of corresponding three-dimensional crystals or other objects. Note the difference in shape between this promorph (rectangle, rectangular amphitect tetragon) and that of the crystal (amphitect octagon) of which it is the promorph.
As noted earlier (previous document), also the above promorph (Autopola Orthostaura Tetraphragma interradialia) can be expressed in a more general way than was done in the previous Figure, to express the possible non-equality (but still congruity) of the antimers (only two by two equal, and two by two symmetric). In the previous Figure, which depicted a representation of this promorph, the antimers were (drawn) equal. The more general representation is depicted in the next Figure.
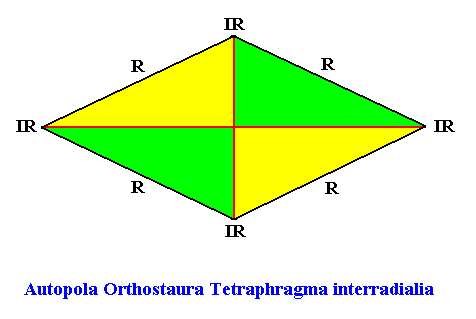
Figure above : Slightly different representation of the promorph (Autopola Orthostaura Tetraphragma interradialia) of the amphitect octagonal crystal with four antimers in interradial configuration. It is now a rhombus (not a rectangle), and expresses the overall non-equality (but still congruity -- two by two equal, and two by two symmetric) of the four antimers (green, yellow) as they are in the above amphitect octagonal crystal. Compare with the representation of the Autopola Orthostaura Tetraphragma radialia as depicted above .
Two antimers.
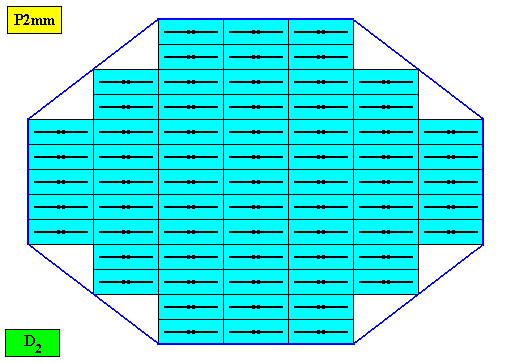
Figure above : An amphitect octagonal D2 two-dimensional crystal. Its D2 motifs (black) have two equal antimers. Microscopic view.
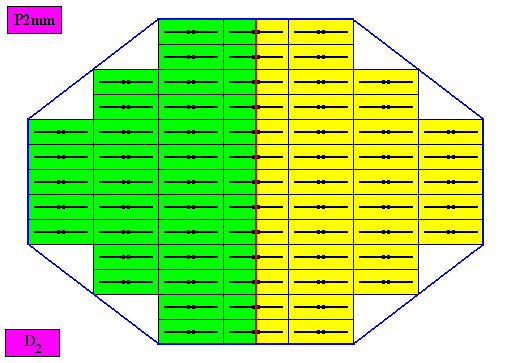
Figure above : The amphitect octagonal D2 two-dimensional crystal of the previous Figure. The case of t w o equal antimers (green, yellow). Note the correspondence between the morphology of the (microscopic) motif (as translation-free residue) and the arrangement of the (macroscopic) antimers of the crystal. In this way the promorph, and in particular the number of antimers is based on the morphology of the translation-free residue of the crystal. This residue is explicitly given in the form of a D2 motif (black) inside each rectangular building block. It is -- or represents -- an atomic configuration such that two antimers can be distinguished in it. The crystal is non-eupromorphic because its intrinsic shape suggests eight antimers, while in fact there are only two of them.
By removing lattice lines and motifs we obtain a macroscopic view of the crystal :
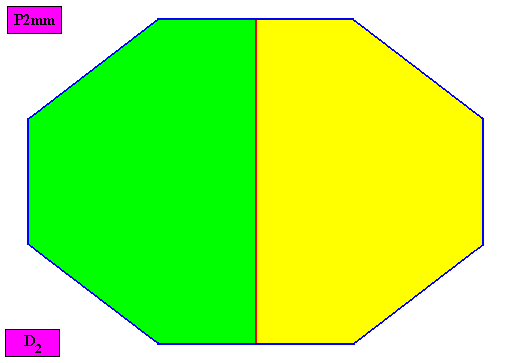
Figure above : Macroscopic view of the amphitect octagonal D2 two-dimensional crystal of the previous Figure with two equal antimers (green, yellow).
The promorph of the above amphitect octagonal non-eupromorphic crystal is (as two-dimensional analogue) belonging to the Autopola Orthostaura diphragma. This promorph is depicted in the next Figure.
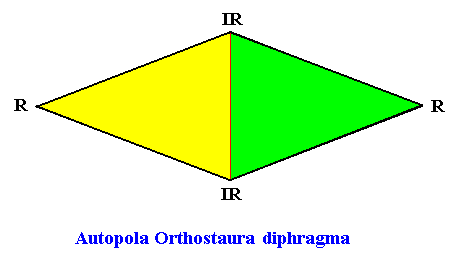
Figure above : The promorph of the amphitect octagonal crystal with two antimers. It is a 2-fold amphitect polygon (rhombus, in the present case -- as '2-fold' -- expressing two antimers) and as such the two-dimensional analogue of the 2-fold rhombic pyramid, which represents the promorph of corresponding three-dimensional crystals or other objects. Note the difference in shape between this promorph (rhombus, amphitect tetragon) and that of the crystal (amphitect octagon) of which it is the promorph.
In the next document we will discuss the fifth crystal shape of our list (as given in Part XVI ) , viz. the bi-isosceles trapezium, with respect to intrinsic symmetry and promorph in holomorphic two-dimensional crystals having this shape intrinsically.
e-mail : 
To continue click HERE for further study of the Theory of Layers, Part XX.