2-D patterns XIX

The Symmetry of Two-dimensional Patterns

As prelude to the symmetry of three-dimensional crystals and organisms

Subpatterns and Subgroups Part XIX (Subgroups and Antisymmetry in Ornaments)

e-mail :

Sequel to Group Theory

As always, we start with reminding the reader about the "Important Remark" near the end of Part III of Group Theory (To see it, click HERE and then go to (end of) Part III ), a Remark concerning the direction of reading products of group elements, like, say, apq. We read such products (from that Remark onwards) from back to front. Thus (with respect to apq) first q, then p, and then a.

Infinite two-dimensional periodic patterns, or Ornaments (sequel)

In the previous document we considered antisymmetry patterns derivable from a P2gg pattern. Here we will comtinue this, before going on to other plane groups.

An antisymmetry pattern can be derived from the P2gg pattern of Figure 9 of Part XVII of the present Series by replacing both generating glide reflections by the corresponding antisymmetry transformations e₁g_h and e₁g_v . Again, the antiidentity transformation e₁ stands for the color permutation (Blue Red) (cycle notation) with respect to the background color. The next Figures show the derivation in several steps.

Figure 1. From the identity element a vertical and horizontal row of elements, alternating with respect to color, are generated by the two antisymmetry transformations e₁g_h and e₁g_v .

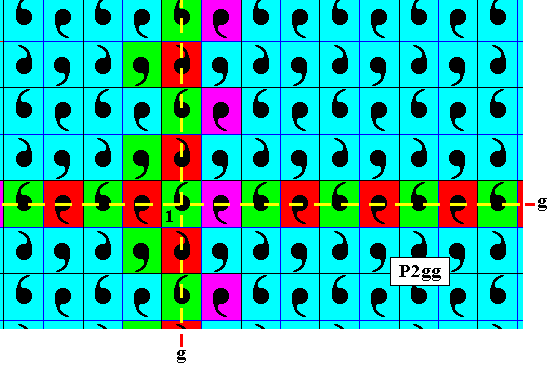
Figure 2. The element directly to the right of the identity element gives rise to a series of new elements alternating with respect to color, in virtue of the antisymmetry transformation e₁g_v .

Figure 3. If we subject all the so far generated elements repeatedly to the horizontal translation the existing color alternation will be repeated in the horizontal direction, resulting in the antisymmetry pattern. As a check on consistency we can consider the following : From the red element a the antisymmetry transformation e₁g_v generates -- among others -- two new elements, b and c , which are -- as they should be -- blue (indicated, for the time being, by green). And these two elements b and c also relate to each other by a vertical translation, implying that they should be of the same color, which indeed they are.
Further, two times applying the transformation e₁g_v to the identity element is the same as applying a vertical translation to the identity element (e₁g_ve₁g_v = g_v² = t_v), and in both cases there should be no color change involved, so in the vertical row containing the identity element, we expect to see vertical translations without color change, which indeed is the case.

If we now restore purple to red, and green to blue, we get our antisymmetry pattern :

Figure 4. Antisymmetry pattern derived from the P2gg pattern of Figure 9 of Part XVII of the present Series by replacing both generating glide reflections by their corresponding antisymmetry transformations. The result is a checkerboard-like pattern of alternating background colors.

The set of blue elements (containing the identity element) forms the subgroup P2. To see this we first isolate this subgroup :

Figure 5. Isolated subgroup (represented by the pattern of commas) of the antisymmetry pattern of Figure 4.

By inserting the underlying lattice, we can clearly see that the above subgroup has P2 symmetry :

Figure 6. Isolated subgroup (represented by the pattern of commas) of the antisymmetry pattern of Figure 4. Lattice of the pattern, representing this subgroup, indicated, and demonstrating the P2 symmetry of the pattern. Each mesh contains two commas related to each other by a half-turn. When we start with the (area representing the) identity element, we can generate a second element by the generating half-turn [as it is -- as a 2-fold rotation axis -- present in the center of the unit mesh containing the identity element, and which is also an element of the full (antisymmetry / generating group)], and if we then multiply the two elements according to the vertical and horizontal generating translations, we get the whole pattern representing the subpattern of blue elements, proving that the latter subpattern is a s u b g r o u p of the antisymmetry pattern as well as of the generating P2gg symmetry pattern.

Because the involved subgroup has P2 symmetry, the symbol of the corresponding antisymmetry group must read P2gg / P2 .

The point lattice underlying the above derived antisymmetry pattern is indicated in the next Figure.

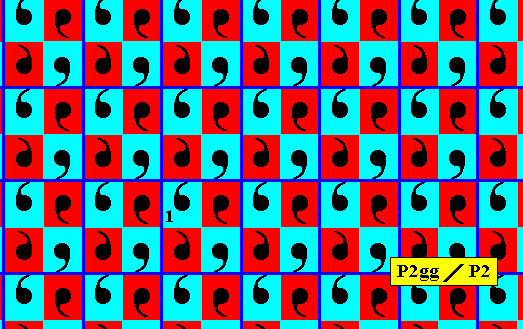
Figure 7. Point lattice (indicated by dark blue connection lines) of the above derived antisymmetry pattern P2gg / P2 . Compare with next Figure.

Figure 8. Point lattice (indicated by red connection lines) of the generating P2gg symmetry pattern.

Another antisymmetry pattern can be derived from the generating P2gg pattern of Figure 9 of Part XVII of the present Series by replacing both generating translations, t_h and t_v , by their corresponding antisymmetry transformations e₁t_h and e₁t_v , where the antiidentity transformation e₁ is again interpreted as the color permutation (Blue Red) with respect to the background color. Recall that e₁S = Se₁ for any symmetry transformation S , and that e₁² = 1 .
As always, the areas containing background color and motif represent group elements, and are just called "elements".
We will derive the antisymmetry pattern in several steps. Newly generated red elements will, for the time being, be indicated by the color purple, that will be changed to red at the next derivation step. Newly generated blue elements will be colored green, and later be changed to blue.

Figure 9. From the identity element the transformations e₁t_h and e₁t_v generate vertically and horizontally elements with alternating colors.

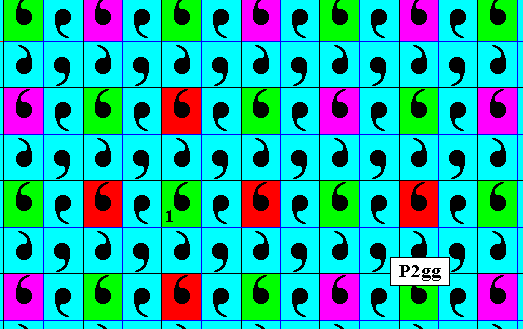
Figure 10. Continuing to apply the transformations e₁t_h and e₁t_v to already generated elements, generates additional red (purple) and blue (green) elements.

Figure 11. Applying the vertical generating glide reflection to the identity element does not involve color change, and gives rise to the element directly above the identity element.
Applying the horizontal generating glide reflection to the identity element also does not involve color change, and gives rise to the element directly to the right of the identity element. For the generating glide reflections see Figure 10 of Part XVII of the present Series. We must, however, realize that in fact we can choose the color of both new elements to be blue or red, because the vertical and horizontal glide reflections are not independent of the vertical and horizontal translations (which are now antisymmetry translations), in virtue of the fact that two times a glide reflection is equal to a translation. An in the present case the translations -- as e₁t_v and e₁t_h -- involve color changes. We will elaborate on this below.

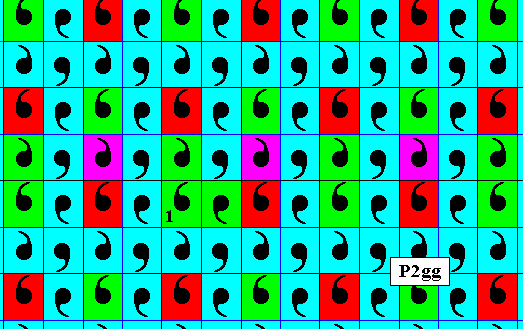
Figure 12. Applying the transformation e₁t_h to the element just above the identity element, gives rise to still more red (purple) and blue (green) elements, as indicated.

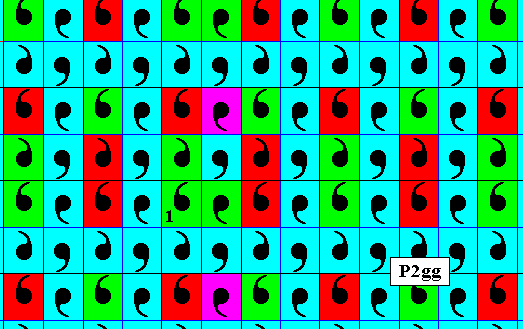
Figure 13. Applying the transformation e₁t_v to the element just to the right of the identity element, gives rise to still more red (purple) and blue (green) elements, as indicated.

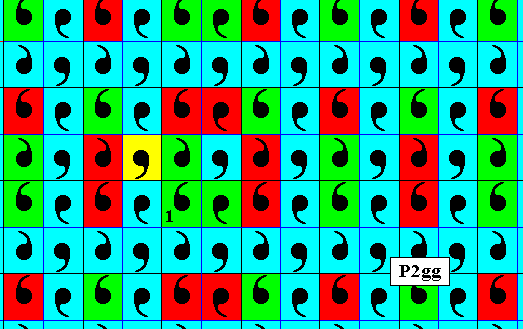
Figure 14. Applying the generating vertical glide reflection to the element just to the right of the identity element, gives rise to a new blue element ( b l u e -- but indicated by yellow -- because it is the element g_vg_h , namely the result of first applying the generating horizontal glide reflection to the identity element and then, on the result, applying the generating vertical glide reflection, while, evidently, there is no e₁ involved). We must, however, realize that in this case -- and in all similar cases gone before -- the new element can also be (set as) red, because the vertical glide reflection is not independent from the vertical translation, and the latter -- as e₁t_v -- involves color change. We shall elaborate on this further down.

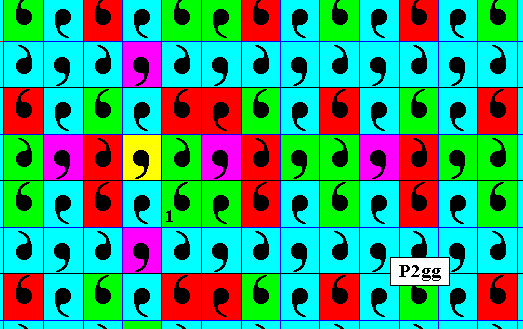
Figure 14a. Applying the transformations e₁t_v and e₁t_h to the above derived element g_vg_h , gives rise to still more red (purple) and blue (green) elements.

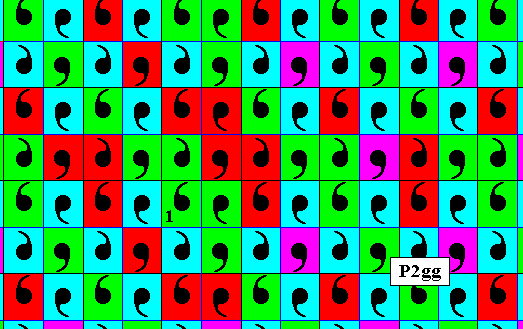
Figure 15. Applying the transformation e₁t_h to all the already generated elements results in still more red (purple) and blue (green) elements.

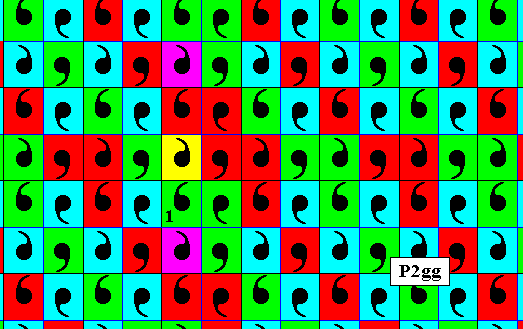
Figure 16. Applying the transformation e₁t_v to the blue element just above the identity element (here indicated by yellow) results in some more elements.

Figure 17. The bottom line is filled in by applying the transformation e₁t_h .

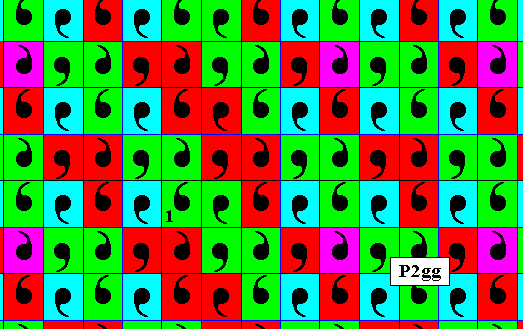
Figure 18. Applying the transformation e₁t_v to all the already generated elements results in still more new elements.

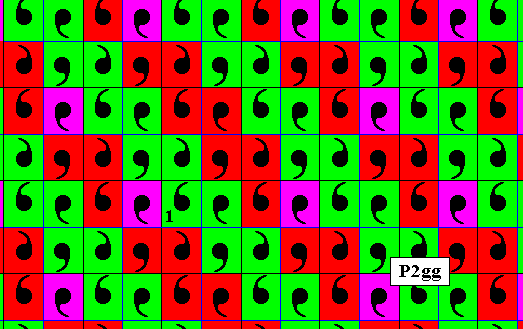
Figure 19. Applying the transformation e₁t_h to all the already generated elements results in all the remaining elements of the visible part of the pattern (which itself is infinite) filled in.

Restoring the colors gives the following antisymmetry pattern :

Figure 20. Antisymmetry pattern derived from the generating P2gg pattern of Figure 9 of Part XVII of the present Series by replacing both generating translations, t_h and t_v , by their corresponding antisymmetry transformations e₁t_h and e₁t_v .

Let's dwell a little longer on the just derived antisymmetry pattern.

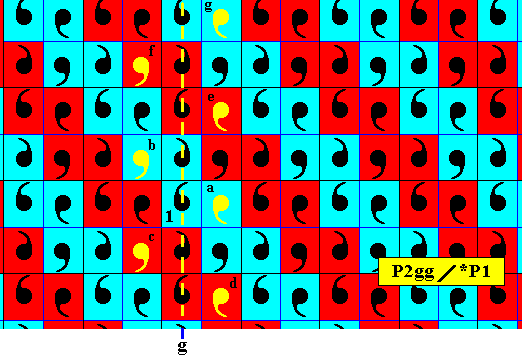
Figure 21. The vertical generating glide line (dashed line) and some of its effects indicated.
At first sight we seem to detect a contradiction : If we subject the blue element a to the vertical generating glide reflection in the upward direction, we get the blue element b , as was to be expected, because the vertical glide is not an antisymmetry transformation. But if we subject the element a to that same glide reflection but now in the downward direction, we get the red element c , despite the fact that this glide reflection is -- as has been said -- not an antisymmetry transformation.
We must, however, realize that a vertical glide reflection is not independent of a vertical translation, because two consecutive vertical glide reflections together form a vertical translation, and the latter is in the present case an antisymmetry transformation, that effects alternating colors.
A glide reflection has as its symmetry element a glide line. Not any p o i n t has a particular status with respect to the glide reflection. So we cannot say that our generating vertical glide reflection begins at, say, the element a (and then, when repeated, extends upwards as well as downwards). It is more appropriate to say that it starts at i n f i n i t y , either from above or from below. Say that it comes from the 'above-infinity' and thus descending all the way down to the 'down-infinity'. Then we see the element g (in the present Figure) being blue. Applying to this element two times a glide reflection downwardly results in the element e , which is red, and this color is to be expected, because two times a vertical glide reflection is equivalent to a vertical translation t_v (which in our case is replaced by the antisymmetry transformation e₁t_v ) . Applying it four times to the blue element g results in the element a , which is also blue, because four times a vertical glide reflection is equivalent to two vertical translations, which here are antisymmetry translations. And two such translations involve two e₁'s , so together do not effect a color change. Six times applying the vertical glide reflection to the element g results in the red element d , because six consecutive glide reflections together constitute three translations. And now, in the corresponding antisymmetry translations, three e₁'s are involved and thus effect a color change with respect to the color of the element g , which is blue, and indeed the element d is red.
The same color alternation can be observed with respect to the elements f (red), b (blue), c (red) .
The upshot of all this is that we have, in deriving the present antisymmetry pattern, not introduced a contradiction. The pattern of the present Figure is indeed a true antisymmetry pattern.

The next Figures give the point lattice of the just derived antisymmetry pattern.

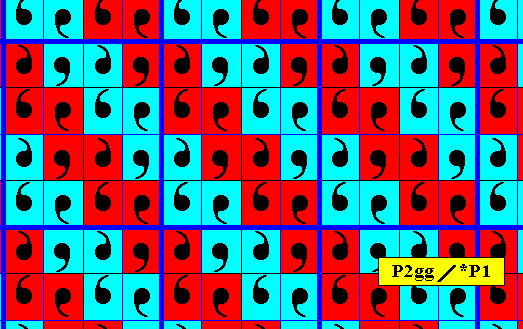
Figure 22. Point lattice (indicated by strong dark blue connection lines) of the above derived antisymmetry pattern (both translations replaced by antisymmetry translations). Compare with the point lattice of the generating P2gg pattern of Figure 8.

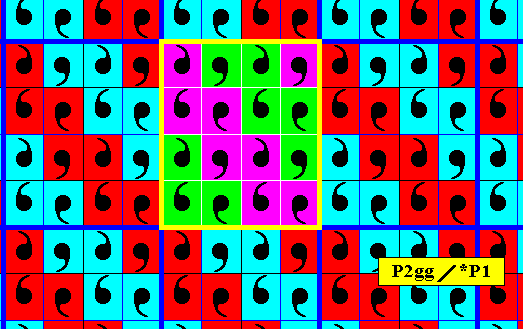
Figure 23. Point lattice (indicated by strong dark blue connection lines) of the above derived antisymmetry pattern. A unit mesh is indicated by yellow outline and alternative colors.

Now we will investigate the subpattern implied by the above antisymmetry pattern, namely the pattern consisting of all the blue areas.

The next Figure isolates the blue elements from the just derived antisymmetry pattern of Figure 20.

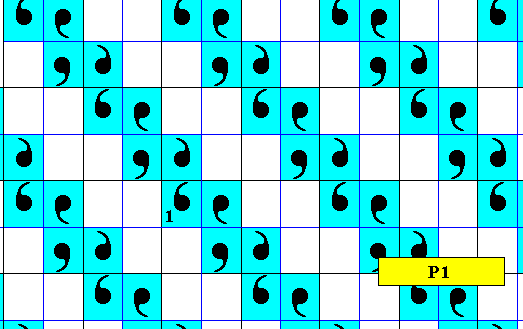
Figure 24. The blue areas of the above derived antisymmetry pattern are isolated.

The next Figure shows the point lattice of the set of blue areas, demonstrating that the pattern is periodic.

Figure 25. Point lattice (indicated by strong red connection lines) of the pattern of blue areas.

If the above subpattern -- which has the structure of a group -- is to represent a s u b g r o u p of its corresponding full groups, represented respectively by the generating P2gg pattern (Figure 9 of Part XVII of the present Series ) and by the antisymmetry pattern P2gg / *P1 just derived from it (Figure 20 ), then all elements of the subpattern, i.e. all blue areas of the pattern of Figure 25, should also be elements of the full groups, and can be generated from the identity element, i.e. from the area (1) representing the identity element (as it also does in the full groups), by symmetry transformations common to the subpattern and the full groups. So if there is (even only) one element, represented by a blue area (in subpattern as well as full groups), that cannot be so generated, then the subpattern is not a subgroup of the mentioned full groups (despite the fact that this subpattern is itself a group).
Well, in the above Figure we can see that from the area (1) originally representing the identity element we cannot generate all of the remaining areas of that particular mesh (we can only generate one such area [see below]), because the transformations needed are not transformations of the whole pattern, i.e. of the whole subpattern of blue areas. The only symmetry transformations of this pattern are horizontal and vertical translations, with lengths equal to the respective sides of a mesh, and two times as long as the translations of the full P2gg pattern, and thus being symmetry transformations also belonging to the latter. When we apply these translations to the area originally representing the identity element we get outside the mesh, so these translations do not generate elements within that mesh. All this means that the areas originally representing group elements do not do so anymore. The whole content of a mesh is now representing a group element of the pattern, which implies that we now have an asymmetric motif -- represented by all the commas in a mesh -- that is translated vertically and horizontally, which in turn means that the symmetry of the pattern is that of the plane group P1 . And because -- as demonstrated below -- at least one area originally representing a group element cannot be generated from the area originally representing the identity element by any transformation of the full group (the plane group P2gg or the antisymmetry group P2gg / P1), the pattern of blue areas is not a subgroup of the full group P2gg, neither is it a subgroup of the antisymmetry pattern derived from it. It is just a subpattern. And in virtue of this fact we place an asterisk in front of its group symbol -- P1 -- when denoting the symbol of the antisymmetry group : P2gg / *P1 .

A pattern that does represent a g e n u i n e s u b g r o u p of the generating P2gg pattern is, for instance, the subgroup P2 which was found earlier (Figure 5 ) in connection with another antisymmetry pattern. Here, from the area originally representing the identity element, all the other elements of the P2 subpattern can be generated by symmetry transformations that belong to the corresponding full groups (the generating P2gg group and the antisymmetry P2gg / P2 group) as well as to the group (P2) represented by the subpattern, namely a half-turn and the horizontal and vertical translations. See next two Figures that concentrate on one of the two full patterns, namely the generating P2gg pattern.

Figure 25a. A pattern representing the P2 subgroup of the P2gg generating pattern (from which antisymmetry patterns were derived).
From the area representing the identity element (of the subpattern as well as of the full pattern), and denoted by 1 , the area representing the element a is generated by a half-turn, the axis of which lies just between those two areas. When we now repeatedly subject these two areas by the translations as indicated, the whole pattern will be generated (A few results of these translations are highlighted (yellow)). The next Figure shows that these symmetry transformations (viz. the half-turn and the two translations) are also elements (symmetry transformations) of the full pattern representing the plane group P2gg .

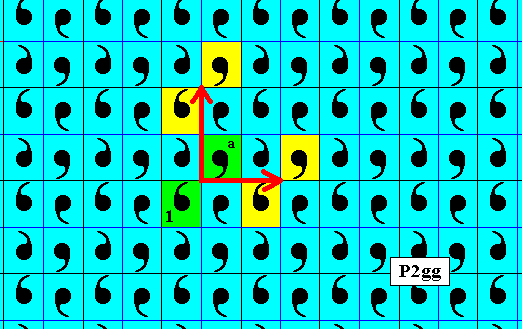
Figure 25b. The generating P2gg pattern of which the P2 pattern of Figure 25a represents a subgroup.
From the area representing the identity element, and denoted by 1 , the area representing the element a is generated by a half-turn, the axis of which lies just between those two areas. When we now repeatedly subject these two areas by the translations as indicated, the above P2 subpattern will be generated. A few results of these translations are highlighted (yellow). These translations as well as the half-turn are symmetry transformations of the full P2gg pattern.

Back to the subpattern involved in our lastly derived antisymmetry pattern.
The next five Figures explain why -- as stated above with respect to Figure 25 -- from the (type of) area originally representing the identity element (of the antisymmetry pattern as well as of the generating pattern) we cannot generate at least some of the other elements of the unit mesh containing that area, by means of symmetry transformations possessed by the subpattern (blue elements) as well as by the antisymmetry pattern and by the generating P2gg pattern. This implies that the present pattern of blue elements, although representing a group (P1), does not have an area representing its identity element which is the same as that in the patterns representing its corresponding full groups (antisymmetry group, generating group). And because a group and any of its subgroups must have the identity element in common, it follows that the present pattern of blue areas is not a subgroup of its corresponding full groups.
The following Figures demonstrate that indeed some of the areas of a given mesh -- areas that should correspond to group elements -- cannot be generated from the supposed identity element by transformations common to this subpattern and the full groups.

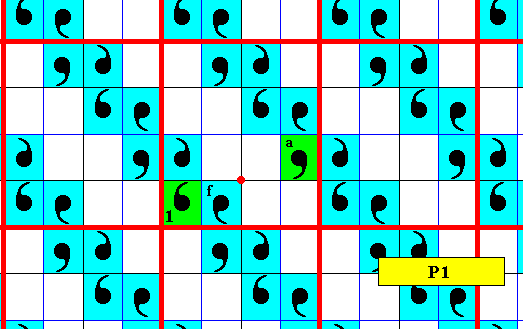
Figure 25c. The 'element' a cannot be generated from the 'identity element' 1 . One could think of a half-turn, the axis of which is indicated by a red dot, but we immediately see that this half-turn is not an element (symmetry transformation) of the whole pattern as such, because the 'element' f does not have an image under this half-turn.

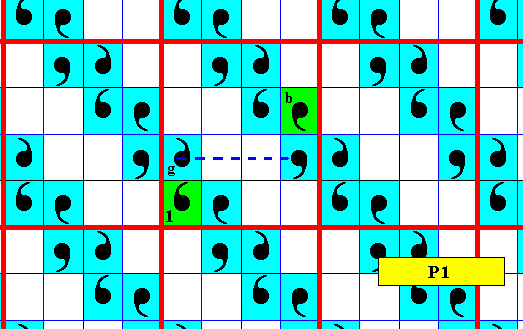
Figure 25d. The 'element' b cannot be generated from the 'identity element' 1 . One could think of a horizontal glide reflection indicated by a dashed line, but we immediately see that this glide reflection is not an element (symmetry transformation) of the whole pattern as such, because the 'element' g does not have an image under two times applying this glide reflection.

Figure 25e. The 'element' c can be generated from the 'identity element' 1 by a translation consisting of the translations t_v (vertical translation) and t_h (horizontal translation) as indicated, i.e. the element c is generated from the element 1 by applying to the latter the symmetry transformation t_ht_v . This latter translation (as well as its two components) are also symmetry transformations of the full P2gg group (See Figure 25b ), and the fact that the components themselves, viz. t_v and t_h , are not elements (symmetry transformations) of the subpattern, does not, in itself, forbid this subpattern to be a subgroup of the P2gg pattern (t_ht_v is common to the subpattern and full pattern).

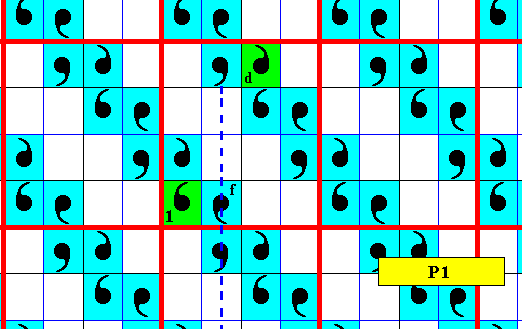
Figure 25f. The 'element' d cannot be generated from the 'identity element' 1 . One could think of a vertical glide reflection as indicated (dashed line), but we immediately see that this glide reflection is not an element (symmetry transformation) of the whole pattern as such, because the 'element' f does not have an image under this glide reflection in the downward direction, neither does the element 1 .

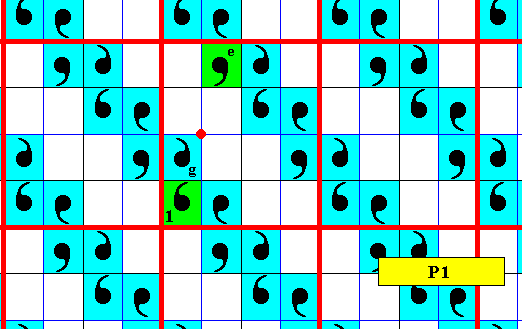
Figure 25g. The 'element' e cannot be generated from the 'identity element' 1 . One could think of a half-turn as indicated (red dot), but we immediately see that this half-turn is not an element (symmetry transformation) of the whole pattern as such, because the 'element' g does not have an image under this half-turn.

There are thus at least some (areas representing) 'elements' (as they are conceived in the pattern representing the full group -- See for instance Figure 25b ) that cannot be generated from the 'element' 1 (again as conceived in the pattern representing the full group) by symmetry transformations that are commonly possessed by the subpattern and the full pattern.
So indeed the pattern of blue elements, although being a group (having P1 symmetry), is not a s u b g r o u p of its corresponding full groups (represented respectively by the above derived antisymmetry pattern (Figure 20 ) and by the generating P2gg pattern (Figure 9 of Part XVII of the present Series ).

The next Figure again depicts our pattern of blue elements but now without all the lines within the meshes in order to let the asymmetric motif in each cell clearly stand out.

Figure 26. Point lattice (indicated by strong red connection lines) of the pattern of blue areas. All the superfluous lines erased.

Alternative antisymmetry pattern

During the derivation of the above antisymmetry pattern (as depicted in Figure 20 ) there were several occasions that allowed us to make a choice of how to proceed further in the derivation. For example already in the very beginning, namely in Figure 11 , we considered the elements that can be generated from the identity element by one time applying respectively the generating vertical and horizontal glide reflections, resulting in one element directly above the identity element, and one to the right of it. The glide lines corresponding to these glide reflections both go through the identity element. Because these glide reflections are not replaced by their corresponding antisymmetry transformations they should not involve color change. So we established the color of the two new elements (as has been said, one directly to the right of the identity element and one directly above the identity element) to be blue (indicated for the time being by the color green), i.e. we established their color to be the same as that of the identity element. But we know that the vertical and horizontal glide reflections are not independent from the respective vertical and horizontal translation. And in the present case these translations appear as antisymmetry translations and thus involve color change. Consequently the vertical and horizontal glide reflections will involve color change : When such a glide reflection is applied two times we get a translation. It is therefore legitimate having the colors of the new elements, generated by one time applying these glide reflections to the identity element, changed, and thus becoming red instead of blue. In this case the single glide reflection transformation involved (either of the vertical or of the horizontal glide reflection) can be seen as a second glide reflection with respect to a first one, or in other words we can interpret the element directly above the identity element (and also the one to its right) as the result of already a second application of a glide reflection transformation. See next Figure.

Figure 27. The vertical and horizontal glide reflections (the glide lines of which go through the identity element and are perpendicular to each other) applied to the identity element generate one element directly above the identity element and one element directly to the right of the identity element. The color of these new elements could be either blue or red. In the present Figure they are given as red (indicated for the time being by the color purple).

In the next Figure we choose the element directly above the identity element to be red (purple) and the element directly to the right of it blue (green). And this choice not only differes from the one in the previous Figure, but also from the one we have made earlier -- in Figure 11 -- during the derivation of the antisymmetry pattern.

Figure 28. In the process of the derivation of the antisymmetry group we let the element directly above the identity element be red (purple). It is generated by applying the vertical glide reflection to the identity element, and we let the element directly to the right of the identity element remain blue (green). It is generated by applying the horizontal glide reflection to the identity element. From here we proceed further with the derivation of the antisymmetry pattern, where both translations are replaced by their corresponding antisymmetry translations e₁t_v and e₁t_h .

Figure 29. Filling in the effect of the antisymmetry translations results in more new elements, blue (green) and red (purple).

As in the earlier derivation (Figure 14 ) we consider applying the vertical generating glide reflection (in the upward direction) to the blue (green) element directly to the right of the identity element. The new element resulting from this is indicated by yellow in the next Figure.

Figure 30. The vertical generating glide reflection (the glide line of which is vertical and goes through the identity element) applied to the blue (green) element directly to the right of the identity element yields a new element indicated by yellow.
Also here we have two choices as to whether the color will be blue or red. We choose blue (green) as we did in the earlier derivation. See next Figure.

Figure 31. The element derived above is set to be blue (green), and the effects of the vertical and horizontal antisymmetry translations is indicated.

Filling now in the effects of the horizontal and vertical antisymmetry translations on all elements so far generated, results in the following antisymmetry pattern.

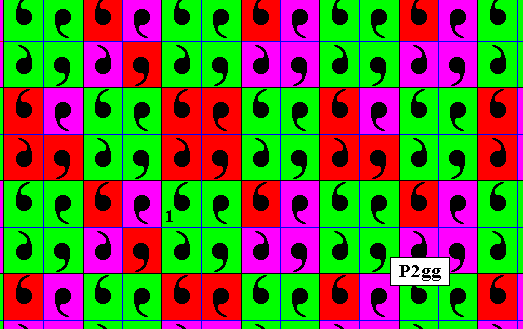
Figure 32. Penultimate stage of the derivation of the antisymmetry pattern.

Restoring the colors, i.e. restoring green to blue and purple to red, gives the definitive antisymmetry pattern :

Figure 33. Final stage of the derivation of the antisymmetry pattern. It is a checkerboard-like pattern.

So by making the above aternative choice we end up with a different antisymmetry pattern. While the first pattern implied a subpattern that was not a subgroup and had P1 symmetry, the second antisymmetry pattern implies a subpattern that is a subgroup and has P2 symmetry.

Let us analyse the implied subpattern of this second antisymmetry pattern as to its status.

First we isolate the pattern of blue elements of the just derived symmetry pattern :

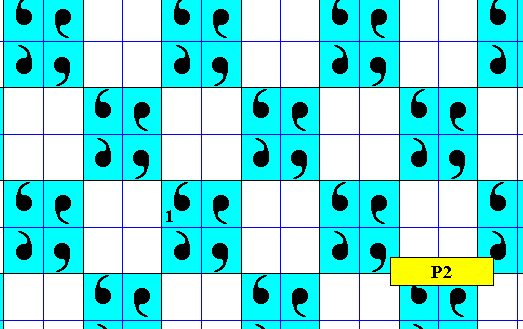
Figure 34. The set of blue areas isolated from the above antisymmetry pattern.
We can see that from all non-translational elements only the half-turn has survived this isolation. The next Figure shows some of these half-turns.

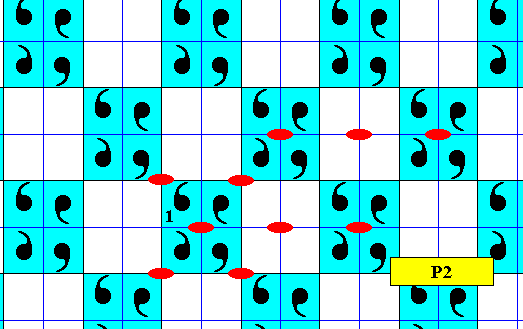
Figure 35. Some half-turns (red solid ellipses) of the isolated pattern of blue areas.

Figure 36. Unit mesh of the above isolated pattern, and its symmetry elements, which are half-turns (blue solid ellipses). This is the fingerprint of P2 symmetry.

The next Figure gives the point lattice of the isolated pattern of blue areas.

Figure 37. Point lattice (indicated by strong red connection lines) of the isolated pattern of blue areas.

The next Figures indicate the point lattice of the aboved derived P2gg / P2 antisymmetry pattern (Figure 33 ).

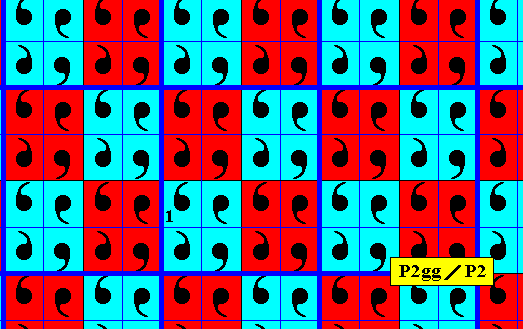
Figure 38. Point lattice (indicated by strong blue connection lines) of the just derived P2gg / P2 antisymmetry pattern.

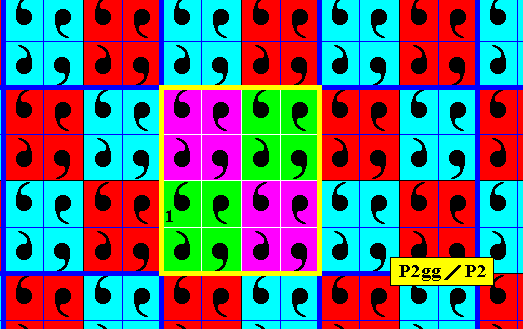
Figure 39. Point lattice (indicated by strong blue connection lines) of the just derived P2gg / P2 antisymmetry pattern. A unit mesh is indicated by yellow outline and alternative colors.

In the next document we will proceed further with the investigation of antisymmetry groups that can be derived from the plane group P2gg.

e-mail :

To continue click HERE for further group theoretic preparation to the study of the structure of three-dimensional crystals (crystallography) and the basic symmetry of organisms (promorphology).

back to homepage

Back to 3-dimensional crystals (conclusion), Organic Tectology and Promorphology

Back to subpatterns and subgroups (introduction)

Back to subpatterns and subgroups Part II

Back to subpatterns and subgroups Part III

Back to subpatterns and subgroups Part IV

Back to subpatterns and subgroups Part V

Back to subpatterns and subgroups Part VI

Back to subpatterns and subgroups Part VII

Back to subpatterns and subgroups Part VIII

Back to subpatterns and subgroups Part IX

Back to subpatterns and subgroups Part X