2-D patterns XXVI

The Symmetry of Two-dimensional Patterns

As prelude to the symmetry of three-dimensional crystals and organisms

Subpatterns and Subgroups Part XXVI (Subgroups and Antisymmetry in Ornaments)

e-mail :

Sequel to Group Theory

As always, we start with reminding the reader about the "Important Remark" near the end of Part III of Group Theory (To see it, click HERE and then go to (end of) Part III ), a Remark concerning the direction of reading products of group elements, like, say, apq. We read such products (from that Remark onwards) from back to front. Thus (with respect to apq) first q, then p, and then a.

Infinite two-dimensional periodic patterns, or Ornaments (sequel)

In the previous document we analysed an antisymmetry pattern as found in Neolithic art, and derivable from a C2mm pattern. Here we will give some more instances from the same period.

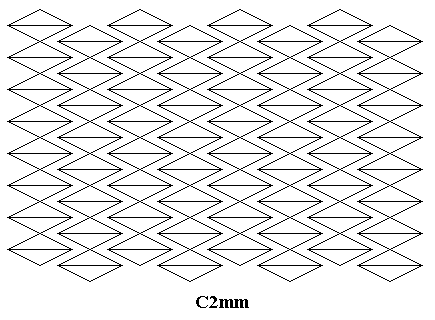
Figure 1. The generating C2mm pattern from which an antisymmetry pattern (Figure 18 ) will be derived that is found in Neolithic art.

Let's analyse this pattern with respect to its symmetry.

Figure 2. Rhombic point lattice (indicated by red connection lines) of the above generating C2mm pattern. For reasons of clarity the pattern is partly colored.

Figure 3. As in previous Figure : Rhombic point lattice (indicated by red connection lines) of the above generating C2mm pattern. A unit mesh is indicated by dark blue outline and alternative colors.

The next Figure shows just the lattice points of the generating C2mm pattern depicted in the previous Figures.

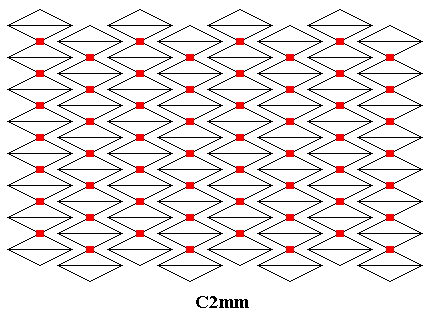
Figure 4. Lattice points (indicated by small red solid rectangles) of the generating C2mm pattern of Figure 1.

The next Figure shows how the same point grid, describing the distribution of equivalent points of the generating C2mm pattern, admits of two different but equivalent lattices, a rhombic and a centered rectangular.

Figure 5. Lattice points (indicated by small red solid rectangles) of the generating C2mm pattern of Figure 1. They admit of two equivalent nets, one with rhombic meshes, the other with centered rectangular meshes.

The next Figure indicates the areas -- within the above generating C2mm pattern -- that can as such r e p r e s e n t group elements, but do not coincide with fundamental regions, because they do not tile the plane without leaving gaps. Nevertheless these areas can serve us well by indicating group elements.

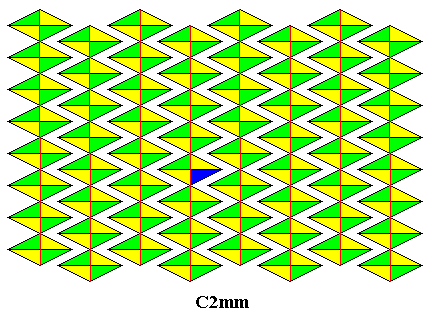
Figure 6. Areas representing group elements (yellow, green) -- without these areas being fundamental regions -- in the generating C2mm pattern of Figure 1 . One such area (dark blue) is chosen to represent the identity element.

The next Figures indicate possible generators of the generating C2mm pattern under investigation.

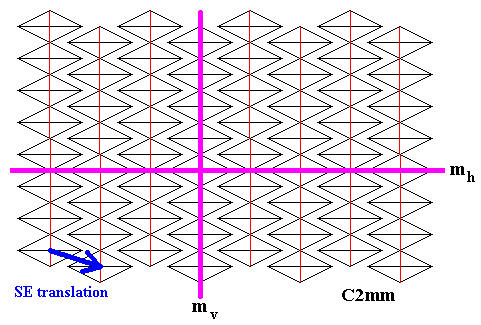
Figure 7. Three generators for the generating C2mm pattern :
Reflection in the line m_h.
Reflection in the line m_v .
South-East translation (Its vector indicated by a blue arrow).
The right-angled triangular areas (four in each rhombus) represent group elements.

Figure 8. Same as previous Figure, but now with coloration (yellow, green) to highlight group elements. Identity element indicated by dark blue.

Now we are ready to derive the antisymmetry ornament, found in Neolithic art, from our generating C2mm pattern of Figure 1 , by replacing the reflection in the horizontal line m_h by its corresponding antisymmetry reflection e₁m_h , where the antiidentity transformation e₁ is interpreted as the color permutation (White Black) (cycle notation) with respect to the color of the interior of the rhombi, and where the initial color is set to be white (We can equivalently say : (e₁ is interpreted as a black-white color permutation with respect to the interior color of the (right-angled triangular) areas assigned to represent group elements).

Reflection in the line m_h involves color change, while the other generators, m_v and the South-East translation t_se do not. The next Figure explains this in detail for eight group elements.

Figure 9. Generation of the first eight group elements of the neolithic antisymmetry ornament :
Application of the antisymmetry transformation e₁m_h to the identity element 1 yields the element e₁m_h . The color white is changed to black.
Application of the symmetry transformation m_v to the just generated element e₁m_h yields the element m_ve₁m_h . The black color remains black (because m_v is not an antisymmetry transformation).
Application of the element m_v to the identity element 1 yields the element m_v . The white color remains white (because m_v is not an antisymmetry transformation).
We now have obtained four group elements (together making up a rhombus).
If we now apply to these elements the South-East translation t_se no color change is involved (as seen from the mentioned four elements).
The next Figures recapitulates all this and proceed further with the generation of new elements of the antisymmetry pattern, ending up with the neolithic antisymmetry ornament.

Generation of the neolithic antisymmetry ornament from the generating C2mm pattern (Figure 1 ) in several steps. The identity element and the newly generated white elements are given, for the time being, as blue. This color will later be restored to white.
In the Figures below, we indicate the generators of the initial C2mm pattern. But of course, the generating horizontal reflection, indicated by m_h , has now become the corresponding antisymmetry reflection e₁m_h :

Figure 10. First phase of the generation of the neolithic antisymmetry ornament from the generating C2mm pattern of Figure 1.

Figure 11. Second phase of the generation of the neolithic antisymmetry ornament from the generating C2mm pattern of Figure 1.

Figure 12. Third phase of the generation of the neolithic antisymmetry ornament from the generating C2mm pattern of Figure 1.

Figure 13. Fourth phase of the generation of the neolithic antisymmetry ornament from the generating C2mm pattern of Figure 1. New elements are obtained by repeatedly applying the South-East translation t_se .

Figure 14. Fifth phase of the generation of the neolithic antisymmetry ornament from the generating C2mm pattern of Figure 1. The elements already present are reflected in the line m_v , not involving a color change (as seen from the mentioned elements).

Figure 15. Sixth phase of the generation of the neolithic antisymmetry ornament from the generating C2mm pattern of Figure 1. Some already obtained elements are repeatedly subjected to the South-East translation (t_se) , yielding still more elements.

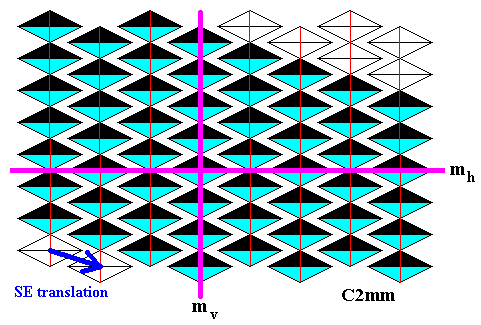
Figure 16. Seventh phase of the generation of the neolithic antisymmetry ornament from the generating C2mm pattern of Figure 1. More elements are generated by repeatedly applying the South-East translation (t_se) to already obtained elements.

Figure 17. Final phase of the generation of the neolithic antisymmetry ornament from the generating C2mm pattern of Figure 1. Because the generated row of elements in Figure 13 extends indefinitely to both sides, the same holds for the row resulting from its reflection in the line m_v (Figure 14 ), and so we can complete the pattern (although it is infinite) by repeatedly applying the South-East translation to the elements of the last mentioned row of elements.

When we now restore blue to white and remove the vertical auxiliary lines indicating borders between left-right adjacent group elements, we get our neolithic antisymmetry ornament :

Figure 18. Antisymmetry ornament from neolithic art, derived from the generating C2mm pattern of Figure 1.
(After JABLAN, 2002)

In order to assess the antisymmetry ornament as to its group theoretic identity, we must identify the subpattern involved in it, namely the set of white elements. Let us isolate this set :

Figure 19. The set of white elements isolated from the above antisymmetry ornament.

Figure 20. Same as previous Figure, but with some coloring for clarity.

Figure 21. Rectangular net underlying the above derived subpattern of the neolithic antisymmetry pattern.

The rectangular net turns out to represent a centered rectangular point lattice :

Figure 22. Centered rectangular point lattice (indicated by blue dots and red connection lines) describing the repetition of motifs of the subpattern derived from the neolithic antisymmetry pattern.

The centered rectangular lattice is equivalent to a rhombic lattice, as the next Figure indicates :

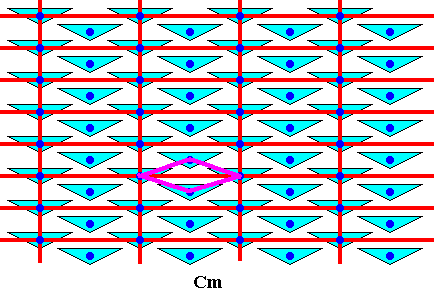
Figure 23. A unit mesh of a rhombic lattice is indicated. The rhombic and centered rectangular lattices are equivalent.

So our subpattern (of the above derived neolithic antisymmetry pattern) consists of motifs with D₁ symmetry (i.e. possessing only one reflection line and no other symmetry transformations), motifs, which are repeated according to a centered rectangular or (equivalently) to a rhombic point pattice, which implies that the symmetry of this subpattern is according to the plane group Cm . Moreover, it is a subgroup of the group represented by the generating C2mm pattern (Figure 1 ) as well as of the group represented by our neolithic antisymmetry pattern (Figure 18 ), because all its elements (including its identity element) are also elements of the two mentioned groups, while the latter have also elements not belonging to the subpattern. Therefore the symbol denoting the antisymmetry group represented by our neolithic antisymmetry pattern must read C2mm / Cm .

In the next document we will show a final example of an antisymmetry pattern from Neolithic art, also derivable from a C2mm pattern.

e-mail :

To continue click HERE for further group theoretic preparation to the study of the structure of three-dimensional crystals (crystallography) and the basic symmetry of organisms (promorphology).

back to homepage

Back to 3-dimensional crystals (conclusion), Organic Tectology and Promorphology

Back to subpatterns and subgroups (introduction)

Back to subpatterns and subgroups Part II

Back to subpatterns and subgroups Part III

Back to subpatterns and subgroups Part IV

Back to subpatterns and subgroups Part V

Back to subpatterns and subgroups Part VI

Back to subpatterns and subgroups Part VII

Back to subpatterns and subgroups Part VIII

Back to subpatterns and subgroups Part IX

Back to subpatterns and subgroups Part X